Work Problems - Civil Service Exam
Overview of Work Problems
Work problems are a category of mathematical questions that deal with rates and the time it takes for workers or machines to complete a task. These problems often require the calculation of how long it takes for two or more parties working together to accomplish a specific job, each working at their own individual rate. Understanding work problems involves grasping a few key concepts:
1. Rate
- Definition: In the context of work problems, the rate refers to the amount of work that can be done per unit of time. It is usually expressed as “task per hour.”
- Example: If John can paint a fence in 6 hours, his rate is 1/6 of the fence per hour.
2. Individual Work Rate
- Definition: This is the rate at which an individual can complete the work alone.
- Example: If Sarah can paint the same fence in 4 hours, her individual work rate is 1/4 of the fence per hour.
3. Combined Work Rate
- Definition: When two or more individuals or machines work together, their rates are added to determine the combined work rate.
- Example: If John and Sarah paint together, their combined work rate is 1/6 + 1/4 = 5/12 of the fence per hour.
4. Total Time Required
- Definition: This is the total amount of time it takes for the combined effort of all involved parties to complete the entire task. It is calculated by taking the reciprocal of the combined work rate.
- Example: For John and Sarah, the total time required to paint the fence together is 1 / (5/12) = 12/5 or 2.4 hours.
Solving Work Problems
To solve a work problem, follow these steps:
- Determine the rate at which each individual can complete the work alone.
- Calculate the combined work rate by adding the individual rates.
- Find the total time required by taking the reciprocal of the combined work rate.
Practice Test on Work Problems - Civil Service Exam
Sample Work Problem:
Problem: Anna and Brian are both tasked with assembling gift boxes for a community event. Anna can assemble all the required gift boxes in 5 hours working alone, while Brian can do the same task in 3 hours working alone. If they work together, how long will it take them to assemble all the gift boxes?
Solution: To find out how long it will take for Anna and Brian to complete the task together, first determine their individual rates of work and then add these rates together to find their combined rate.
Anna’s Rate: If Anna can complete the task in 5 hours, her work rate is:
Rate of Anna = 1 task/5 hours = 1/5 task per hourBrian’s Rate: If Brian can complete the task in 3 hours, his work rate is:
Rate of Brian = 1 task/3 hours = 1/3 task per hourCombined Rate: Add their rates together to find the rate at which they can complete the task together:
Combined Rate=1/5 + 1/3 = 3/15 + 5/15 = 8/15 task per hourTime Required Together: To find out how long it will take them to complete one whole task working together, calculate the reciprocal of their combined rate:
Time required=1/Combined Rate = 1/8/15 = 15/8 hours15/8 hours=1 hour and 52.5 minutes
Conclusion: Anna and Brian will be able to complete the task together in approximately 1 hour and 52.5 minutes.
Problem 2
Problem: Carla can paint a room by herself in 6 hours. Her friend, Jeff, can paint the same room in 4 hours. If they decide to paint the room together while working at their respective paces, how long will it take them to paint the room?
Solution: To solve this, first determine each person’s rate of work, then combine these rates to find the total rate when they work together.
Carla’s Rate: If Carla can complete the task in 6 hours:
Rate of Carla = 1 room/6 hours = 1/6 room per hourJeff’s Rate: If Jeff can complete the task in 4 hours:
Rate of Jeff = 1 room/4 hours = 1/4 room per hourCombined Rate: Add their rates together to find the rate at which they can complete the room together:
Combined Rate=1/6 + 1/4 = 2/12 + 3/12 = 5/12 room per hourTime Required Together: To find out how long it will take them to complete one whole room working together, calculate the reciprocal of their combined rate:
Time required = 1/Combined Rate = 1/5/12 = 12/5 hours = 2.4 hours or 2 hours and 24 minutes
Conclusion: Carla and Jeff will be able to complete the painting of the room together in approximately 2 hours and 24 minutes.
Problem 3.
Problem: Lucy can fill a swimming pool using her hose in 5 hours. Her neighbor, Tim, has a more powerful hose and can fill the same pool in 3 hours. If they both use their hoses to fill the pool at the same time, how long will it take to fill the pool?
Solution: First, calculate each person’s rate of filling the pool, then determine the combined rate.
Lucy’s Rate:
Rate of Lucy = 1 pool/5 hours = 1/5 pool per hourTim’s Rate:
Rate of Tim = 1 pool/3 hours = 1/3 pool per hourCombined Rate: Sum up their rates to get the total filling rate:
Combined Rate = 1/5 + 1/3 = 3/15 + 5/15 = 8/15 pool per hourTime Required Together: Find the reciprocal of their combined rate:
Time required = 1/Combined Rate = 1/8/15 = 15/8 hours = 1.875 hours or 1 hour and 52.5 minutes
Conclusion: Using both hoses, Lucy and Tim can fill the pool together in approximately 1 hour and 52.5 minutes.
Problem 4
A photocopier makes y copies in k hours and 45 minutes. What is its average operating speed in copies per hour?
Choices:
- A. 4𝑦/4𝑘+3 copies per hour
- B. 𝑦/𝑘+0.75 copies per hour
- C. 𝑦/4𝑘+3 copies per hour
- D. 4y copies per hour
Answer: B. 𝑦/𝑘+0.75 copies per hour
Solution:
Convert 45 minutes to hours, which is 0.75 hours. Thus, the total time is 𝑘+0.75k+0.75 hours. The average speed is calculated as 𝑦 copies/𝑘+0.75 hours.
Problem 5.
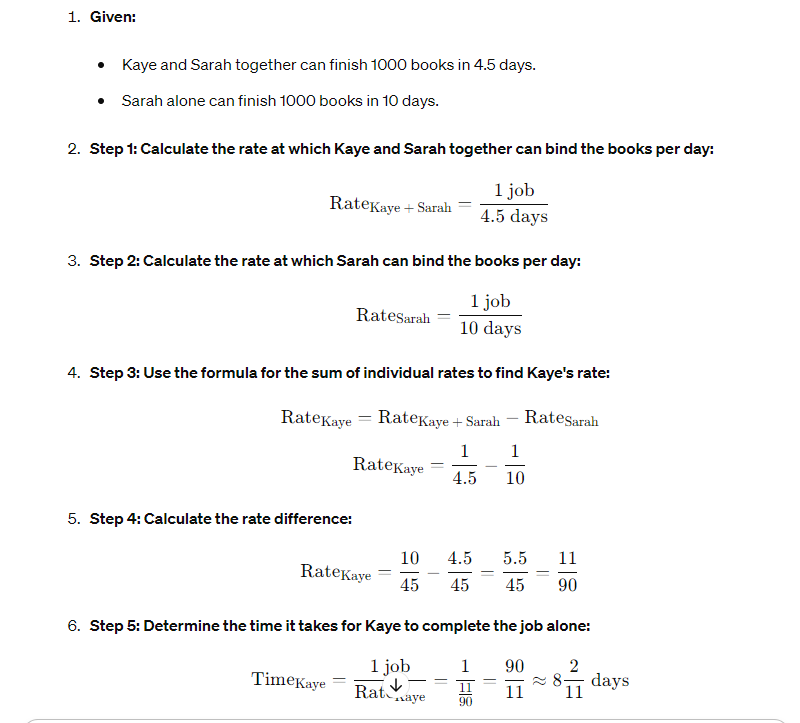
Sarah and Kaye, working together, can finish binding 1000 books in 4 ½ days. Sarah working alone, can bind the 1000 books in 10 days. How long will it take Kaye to do the same job working alone?
Choices:
A. 6 2/11days
B. 7 2/11 days
C. 8 2/11 days
D. 9 2/11 days
Given:
- Sarah and Kaye together can finish 1000 books in 4.5 days.
- Sarah alone can finish 1000 books in 10 days.
Step 1: Calculate the rate at which Sarah and Kaye together can bind the books per day:
Rate Sarah + Kaye=1 job/4.5 days Step 2: Calculate the rate at which Sarah can bind the books per day:
Rate Sarah = 1 job/10 days Step 3: Use the formula for the sum of individual rates to find Kaye’s rate:
Rate Kaye = Rate Kaye + Sarah − Rate SarahRate Kaye = 1/4.5 – 1/10
Step 4: Calculate the rate difference:
Rate Kaye = 10/45 − 4.5/45 = 5.5/45 = 11/90 Step 5: Determine the time it takes for Kaye to complete the job alone:
Time Kaye = 1/ job Rate Kaye = 1/11/90 = 90/11 ≈ 8 2/11 days
Problem 6
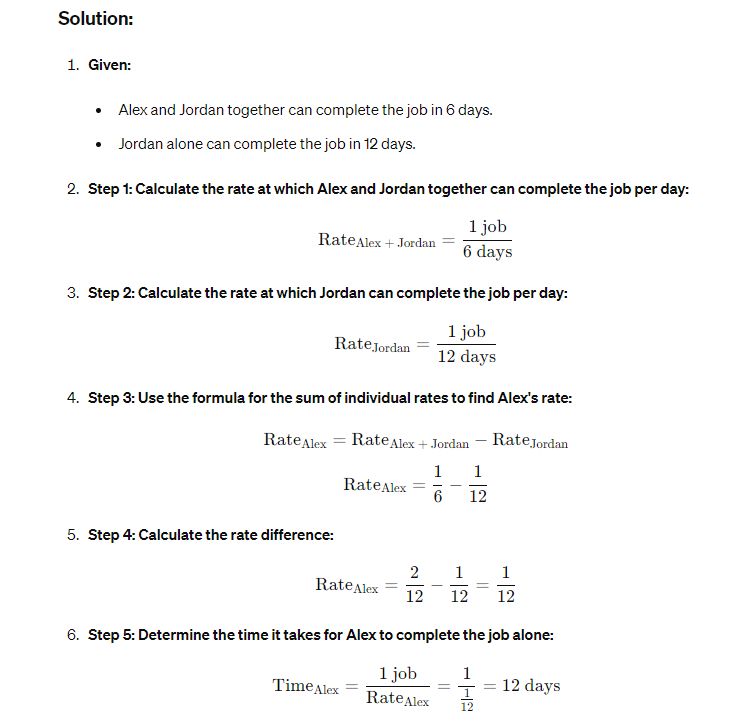
Alex and Jordan, working together, can complete painting a house in 6 days. Jordan alone can complete the same job in 12 days. How long will it take Alex to do the job working alone?
Choices:
- A. 8 days
- B. 9 days
- C. 10 days
- D. 12 days
Answer: D. 12 days